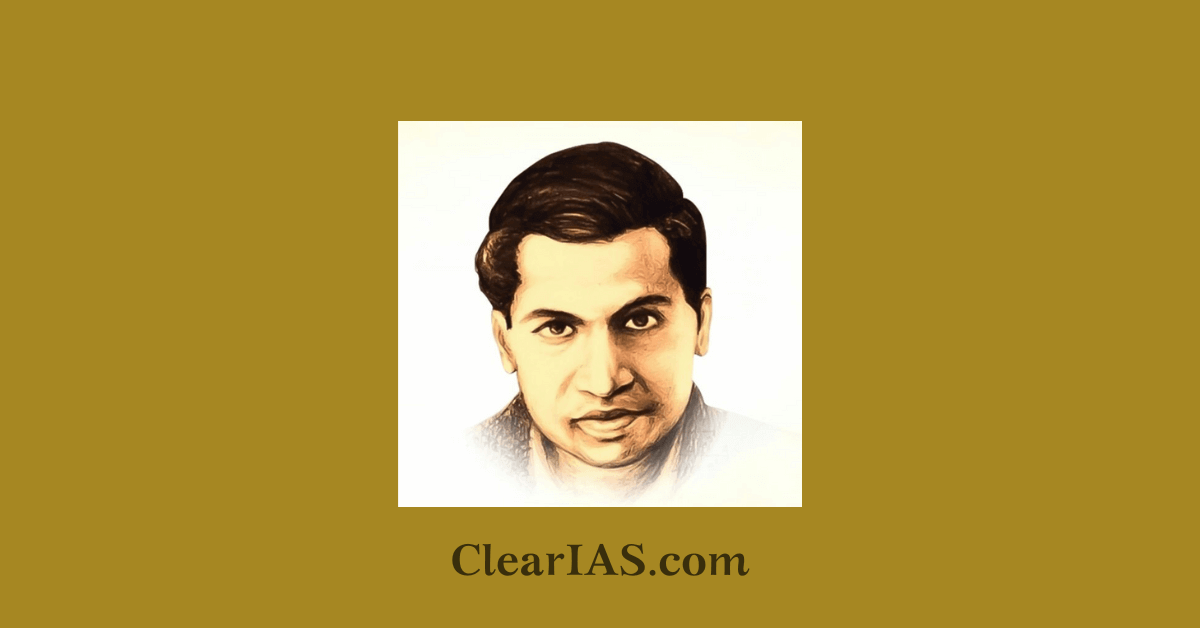
Every year, Srinivasa Ramanujan’s birth anniversary on December 22 is commemorated as National Mathematics Day. Ramanujan made substantial contributions to the analytical theory of numbers and worked on elliptic functions, continued fractions, and infinite series. Read here to know more about his life.
National Mathematics Day is observed annually on December 22nd to mark the anniversary of the eminent mathematician Srinivasa Ramanujan’s birth.
Srinivasa Ramanujan was a self-taught mathematician and one of the most eminent scientists the country has seen.
Ramanujan spent most of his brief but significant life working on theorems that seemed difficult to answer.
His contributions to the fields of elliptic integrals, hypergeometric series, continuous fractions, Riemann series, and functional equations about the zeta function are well known.
The early life of Srinivasa Ramanujan
Ramanujan was born in his grandmother’s house in Erode, a small village about 400 km southwest of Madras (now Chennai).
When Ramanujan was a year old his mother took him to the town of Kumbakonam, about 160 km nearer Madras. His father worked in Kumbakonam as a clerk in a cloth merchant’s shop.
When he was nearly five years old, Ramanujan entered the primary school in Kumbakonam although he would attend several different primary schools before entering the Town High School in Kumbakonam in January 1898.
Before turning 10, he passed his primary examinations in English, Tamil, geography, and arithmetic with the best scores in the district. That year, Ramanujan entered Town Higher Secondary School, where he encountered formal mathematics for the first time.
By age 11 he was recognized as a child prodigy as he had exhausted the mathematical knowledge of two college students who were lodgers at his home.
By age 13, he mastered advanced trigonometry and by age 14 he had started showing affinity to geometry and infinite series.
Ramanujan was shown how to solve cubic equations in 1902. He would later develop his method to solve the quartic. In 1903, he tried to solve the quintic, not knowing that it was impossible to solve with radicals.
He received a scholarship to study at Government Arts College, Kumbakonam. Still, he was so intent on mathematics that he could not focus on any other subjects and failed most of them, losing his scholarship in the process.
He then ran away from his house to Vishakapatnam where he continued his mathematical work, however, and at this time he worked on hypergeometric series and investigated relations between integrals and series. He was to discover later that he had been studying elliptic functions.
In 1906 Ramanujan went to Madras where he entered Pachaiyappa’s College. He aimed to pass the First Arts examination which would allow him to be admitted to the University of Madras. He attended lectures at Pachaiyappa’s College but became ill after three months of study. He took the First Arts examination after having left the course.
- He passed mathematics but failed all his other subjects and therefore failed the examination. This meant that he could not enter the University of Madras.
- In the following years, he worked on mathematics developing his ideas without any help and without any real idea of the then-current research topics other than that provided by Carr’s book.
He became seriously ill again and underwent an operation in April 1909 after which it took him some considerable time to recover.
He married on 14 July 1909 when his mother arranged for him to marry a ten-year-old girl S Janaki Ammal.
The mathematical journey of Srinivasa Ramanujan
Continuing his mathematical work Ramanujan studied continued fractions and divergent series in 1908.
Ramanujan continued to develop his mathematical ideas and began to pose problems and solve problems in the Journal of the Indian Mathematical Society.
- He developed relations between elliptic modular equations in 1910.
- After the publication of a brilliant research paper on Bernoulli numbers in 1911 in the Journal of the Indian Mathematical Society he gained recognition for his work.
Despite his lack of a university education, he was becoming well known in the Madras area as a mathematical genius.
Ramanujan was able to get a job as a clerk in the madras post trust on the recommendation of EW Middlemast, a professor of mathematics in the Presidency college madras.
In January 1913 Ramanujan wrote to G H Hardy having seen a copy of his 1910 book Orders of infinity.
Hardy and Littlewood were very impressed by Ramanujan’s work and helped him get a scholarship at the University of Madras in 1913.
In 1914, Hardy brought Ramanujan to Trinity College, Cambridge to begin an extraordinary collaboration.
Life in Cambridge, England
Right from the beginning, he had problems with his diet. The outbreak of World War I made obtaining special items of food (as he was a strict vegetarian) harder and it was not long before Ramanujan had health problems.
Ramanujan’s collaboration with Hardy led to important results. But his lack of formal education did cause some problems, hence Littlewood was asked to help teach Ramanujan rigorous mathematical methods.
- However, Hardy said- “it was extremely difficult because every time some matter, which it was thought that Ramanujan needed to know, was mentioned, Ramanujan’s response was an avalanche of original ideas which made it almost impossible for Littlewood to persist in his original intention.”
On 16 March 1916 Srinivasa Ramanujan graduated from Cambridge with a Bachelor of Arts in Research (the degree was called a Ph.D. from 1920).
- He had been allowed to enroll in June 1914 despite not having the proper qualifications.
- Ramanujan’s dissertation was on Highly composite numbers and consisted of seven of his papers published in England.
From 1917 to 1918, he fell seriously ill and spent most of his time in various nursing homes.
On 18 February 1918 Ramanujan was elected a fellow of the Cambridge Philosophical Society and then three days later, the greatest honor that he would receive, his name appeared on the list for election as a fellow of the Royal Society of London.
- He had been proposed by an impressive list of mathematicians, namely Hardy, MacMahon, Grace, Larmor, Bromwich, Hobson, Baker, Littlewood, Nicholson, Young, Whittaker, Forsyth, and Whitehead.
- His election as a fellow of the Royal Society was confirmed on 2 May 1918, then on 10 October 1918, he was elected a Fellow of Trinity College Cambridge, the fellowship to run for six years.
He returned to India in 1919.
He died in 1920 at the age of 32 due to ill health.
Ramanujan’s work in mathematics
The letters Ramanujan wrote to Hardy in 1913 contained many fascinating results.
- Ramanujan worked out the Riemann series, the elliptic integrals, the hypergeometric series, and the functional equations of the zeta function.
- On the other hand, he had only a vague idea of what constitutes mathematical proof.
- Despite many brilliant results, some of his theorems on prime numbers were completely wrong.
Ramanujan independently discovered the results of Gauss, Kummer, and others on hypergeometric series. Ramanujan’s work on partial sums and products of hypergeometric series has led to a major development in the topic.
Ramanujan left several unpublished notebooks filled with theorems that mathematicians have continued to study.
G N Watson, Mason Professor of Pure Mathematics at Birmingham from 1918 to 1951 published 14 papers under the general title Theorems stated by Ramanujan, and in all, he published nearly 30 papers that were inspired by Ramanujan’s work.
Hardy passed on to Watson a large number of manuscripts of Ramanujan that he had, both written before 1914 and some written in Ramanujan’s last year in India before his death.
The number 1729 is known as the Hardy–Ramanujan number after a famous visit by Hardy to see Ramanujan at a hospital.
- In Ramanujan’s words, “It is a very interesting number; it is the smallest number expressible as the sum of two cubes in two different ways.”
In his obituary of Ramanujan, written for Nature in 1920, Hardy observed that Ramanujan’s work primarily involved fields less known even among other pure mathematicians.
Legacy of Srinivasa Ramanujan
The year after his death, Nature listed Ramanujan among other distinguished scientists and mathematicians on a “Calendar of Scientific Pioneers” who had achieved eminence.
Ramanujan’s home state of Tamil Nadu celebrates 22 December (Ramanujan’s birthday) as ‘State IT Day’.
Stamps picturing Ramanujan were issued by the government of India in 1962, 2011, 2012, and 2016.
In 2012, former Prime Minister Dr. Manmohan Singh paid tribute to the mathematician during a ceremony in Chennai to commemorate Ramanujan’s birth anniversary and declared December 22 National Mathematics Day.
The Ramanujan Math Park located in Kuppam, Andhra Pradesh, was inaugurated on this day in 2017.
-Article written by Swathi Satish





Leave a Reply